The rule for multiplying fractions says to multiply across the numerators, (top of the fractions), and also multiply across the denominators, (bottom of the fractions).

Then always remember to state your answers in lowest terms, (which means reduce them to the smallest equal fraction or mixed number)

EX:

*Actually, you are allowed to do the reducing BEFORE you multiply if there is a number that will divide out of the top and the bottom sections.

For example in the problem above, I see that there is a 4 being multiplied in the top and in the bottom sections of the problem.  Since I know that 4/4 = 1, this is a Common Factor that I can divide out now instead of at the end. Doing this leaves me with the problem,
3 x 1 = ...........but wait there is something else hidden this problem
1....9

Do you see that you can also divide a 3 from the top and from the bottom at this point?

If we see the common factor of 3 and divide it out of the numerator in the fist fraction and divide it out of the denominator in the second fraction we get.

1 x 1 = 1
1....3....3

A rather interesting aspect of fraction multiplication is that you can DRAW fraction multiplication.

All you need is a square.

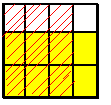
Now let's say we want to illustrate 3/4 of 2/3, (that means 3/4 x 2/3). We just take our square, and cut it into thirds and shade 2 of those thirds.  

Now we cut the same square into FOURTHS using VERTICAL slices and we shade 3 of those fourths.

Now to truly SEE the answer to 3/4 of 2/3 all you need do is look for the overlap. You see the yellow part is two thirds of the whole square, and we cut that yellow part into fourths and we shaded 3 of those fourths.  The red shaded yellow section consists of   6 rectangles out of the entire 12 rectangles in the square. 

In other words, multiplying across the numerators gives you the number of overlapped sections and multiplying across the denominators gives the total sections in the square.

Now let's play:

Call up your paint program out of your "accessories" file and try to DRAW the following fraction products. 

Be as creative as you can, and print them out if you like when you are done.(be sure to write the answer on your drawing)

1.) try to draw using a big square 1/2 x 2/3

2.) try to draw using a square 2/3 x 3/4

3.) draw 3/5 x 5/6 using a big square

4.) finally try this one: 7/12 x 7/8